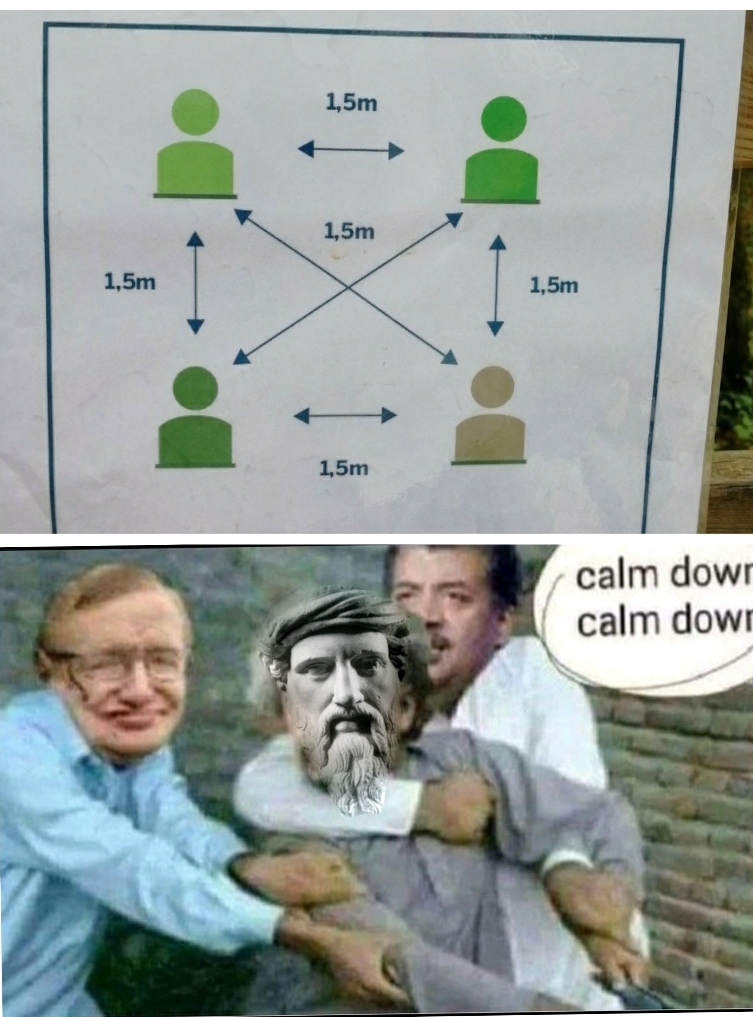
You're all thinking too two-dimensionally. Clearly the people are being instructed to arrange themselves into a tetrahedron.
this post was submitted on 15 May 2025
651 points (98.1% liked)
memes
14865 readers
5504 users here now
Community rules
1. Be civil
No trolling, bigotry or other insulting / annoying behaviour
2. No politics
This is non-politics community. For political memes please go to [email protected]
3. No recent reposts
Check for reposts when posting a meme, you can only repost after 1 month
4. No bots
No bots without the express approval of the mods or the admins
5. No Spam/Ads
No advertisements or spam. This is an instance rule and the only way to live.
A collection of some classic Lemmy memes for your enjoyment
Sister communities
- [email protected] : Star Trek memes, chat and shitposts
- [email protected] : Lemmy Shitposts, anything and everything goes.
- [email protected] : Linux themed memes
- [email protected] : for those who love comic stories.
founded 2 years ago
MODERATORS
Tetrahedrons man, tetrahedrons.
So this makes me wonder if one could force a move into a higher dimension by somehow constraining a set of connected distances in this way.
Sort of like protein folding as a way to bootstrap a dimensional jump.
You might like And He Built a Crooked House by Robert Heinlein - the story of a tesseract-shaped house that folds itself into a real tesseract during an earthquake.
Middle one should be the square root of 4.5 meters, or 2.12 meters
No one said right angles.
Equal sides in a triangle are only possible if the corners are equal. So, 60⁰ each.
But its height cannot be half of base because of the same Pythagorean theorem
(1,5)²+(1,5/2)²=2,8125
sqrt(2,8125) ≈ 1,677, which is half of a diagonal
So, we get 4 sides that are 1,5 in a parallelogram, but diagonals are 1,5 and 3,354, as opposed to both being 1,5 as shown on the picture
TL;DR: Won't work because Pythagorean theorem
It's funny how we say "because of such and such theorem" as if if some greek dude didn't come up with his little story, the height could totally be half of base.
Decolonialize Maths!
We do need short names, but they don't all have to be wyt guys. Pre-globalization, I'm sure many true maths statements were independently discovered by many people.
Pyramid?
Yes, it is possible with a 3-sided pyramid, i.e. tetrahedron. If we dont look at all 4 points as being on the same plane but 2 opposite corners being offset above or below the other two, this could totally be a tatrahedron.
So those two darker green symbols would represent someone shorter or taller. Totally plausible.
Wdym?
They could each be on the vertices of a tetrahedron for all we know...
We do know that, with those measurements, they aren't confined to a single (Euclidean) plane.
Exactly! I regret that I have but one upvote to give.
I was thinking of plane surfaces, but if their altitudes are different, I guess it'd be possible.
ikr? It's like some people don't even recognize a tetrahedron
if the people were aranged in 3d in the shape of a tetrahedron (triangular pyramid) this would work out fine
No
Unless the measurement is from the corner to where the lines cross (peak of the pyramid), but that is not at all clear from how the diagram is drawn.
It's not a square based pyramid, it's a triangular based pyramid. Imagine the top right hand one floating up onto the air and moving to hover above the centre of the other three (which move to make an equalateral triangle). The distances work but the layout changes.
You mean a tetrahedron.
I do.
Explanation:
So, Theres the sentence of Pythagoras. It says that c^2^ = a^2^ + b^2^ when the triangle has a 90° corner
Since a square is just 2 triangles, it applies. That means c (the distance from Person a to Person c) should be √(2×1.90^2^). But that is 2.7m.
These instructions are in 3d.
Even if we would take it from the head of person a to the toe of c, its still a triangle
It's the plan view of 4 equilateral triangles arranged into a tetrahedron. There are no right angles so Pythagoras doesn't apply.
What? Everytime I meet other people we always arange ourselves in the shape of a simplex of the appropriate dimension. Doesn't everyone?
So the fifth person to arrive moves to the centre of the tetrahedron and shifts roughly 1.299m into the past or future.
I have a few questions.
- How do you attain time offset?
- Doesn't that make conversation difficult?
- What even is the fifth dimension?
- How do you convert a distance in metres into a distance in time? You would surely then have a universal m/s? Oh, wait, there is a universal speed, it's the speed of light, which means 1.299m is equivalent to about 4.3 billionths of a second, which is considerably less impressive for question 1 and just not at all problematic for question 2.
- If you're using very fast motion for your time offset, doesn't that make conversation even more difficult? How fast would you need to be going to dilate time for a few billionths of a second? Doesn't Heisenberg uncertainty start to have an impact here? How can you be sure you got it right?
- So, the diagram doesn't represent it well, but the 1.5m is a minimum. So, I just delay myself by half a heartbeat which is well over 4.3^e-9s.
Yes, but if they're just minimums, there's no need for even using the third dimension, let alone the fourth.
Oh, I may have violated distancing protocols then. My personal delay device doesn't have sub-microsecond accuracy. Should I will have gotten a test for time-invariant COVID ?
I won't tell if you won't tell.
If you have to ask, you wouldn't understand.
If I understood, I wouldn't have to ask.
view more: next ›